Податотека:Rolling Racers - Moment of inertia.gif
Rolling_Racers_-_Moment_of_inertia.gif (480 × 270 пиксели, големина: 1,6 МБ, MIME-тип: image/gif, кружно, 126 кадри, 4,2 с)
Напомена: Поради технички ограничувања, минијатурите на GIF-слики со висока разделност како оваа нема да се анимираат.
Оваа податотека е од Ризницата и може да се користи во други проекти. Описот од нејзината описна страница е прикажан подолу.
| ОписRolling Racers - Moment of inertia.gif |
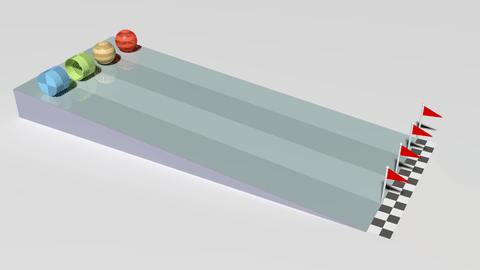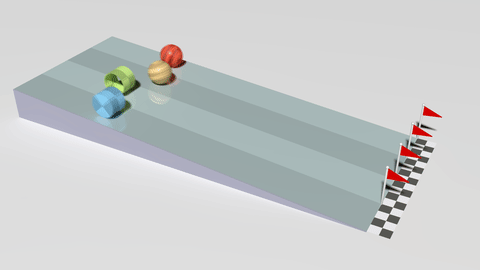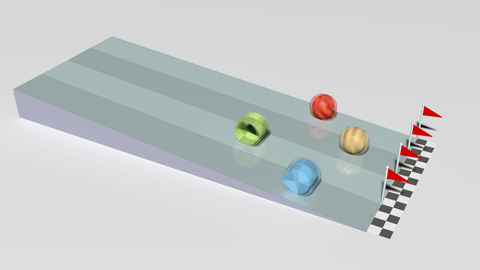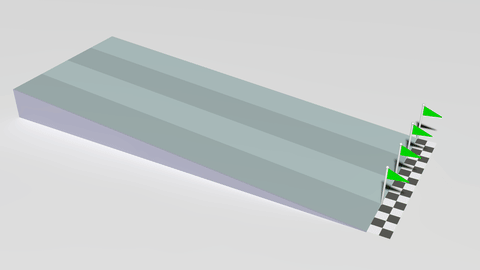
The objects are, from back to front:
At any moment in time, the forces acting on each object will be its weight, the normal force exerted by the plane on the object and the static friction force. As the weight force and the normal force act on a line through each object's center of mass, they result in no net torque. However, the force due to friction acts perpendicular to the contact point, and therefore it does result in a torque, which causes the object to rotate. Since there is no slipping, the object's center of mass will travel with speed Solving for Since the torque is constant we conclude, by Newton's 2nd Law for rotation Where, v0 = 0 and d is the total distance traveled. Therefore, we have: For a ramp with inclination θ, we have sin θ = h / d. Additionally, This final result reveals that, for objects of the same radius, the mass the object are irrelevant and what determines the rate of acceleration is the geometric distribution of their mass, which is represented by the value of k. Additionally, we observe that objects with larger values of k will accelerate more slowly. This is illustrated in the animation. The values of k for each object are, from back to front: 2/3, 2/5, 1, 1/2. As predicted by the formula found above, the solid ball will have a larger acceleration, reaching the finish line first. |
|||
| Датум | ||||
| Извор | сопствено дело | |||
| Автор | Lucas Vieira | |||
| Дозвола (Повторно користење на податотекава) |
|
|||
| Други верзии | OGG Theora Video: small and [[:File:Rolling Racers - Moment of inertia (HD).ogv|large (HD) and for classroom educational purposes a static image of the finish at File:Rolling Racers - Moment of inertia Photofinish.jpg ]] |
POV-Ray source code
Available at the video version's description page.

|
Оваа слика е оценета според критериумите за квалитетни слики и се смета за Квалитетна слика.
العربية ∙ جازايرية ∙ беларуская ∙ беларуская (тарашкевіца) ∙ български ∙ বাংলা ∙ català ∙ čeština ∙ Cymraeg ∙ Deutsch ∙ Schweizer Hochdeutsch ∙ Zazaki ∙ Ελληνικά ∙ English ∙ Esperanto ∙ español ∙ eesti ∙ euskara ∙ فارسی ∙ suomi ∙ français ∙ galego ∙ עברית ∙ हिन्दी ∙ hrvatski ∙ magyar ∙ հայերեն ∙ Bahasa Indonesia ∙ italiano ∙ 日本語 ∙ Jawa ∙ ქართული ∙ кыргызча ∙ 한국어 ∙ kurdî ∙ Latina ∙ Lëtzebuergesch ∙ lietuvių ∙ македонски ∙ മലയാളം ∙ मराठी ∙ Bahasa Melayu ∙ Nederlands ∙ Norfuk / Pitkern ∙ polski ∙ português ∙ português do Brasil ∙ qaraqalpaqsha ∙ rumantsch ∙ română ∙ русский ∙ sicilianu ∙ slovenčina ∙ slovenščina ∙ shqip ∙ српски / srpski ∙ svenska ∙ தமிழ் ∙ తెలుగు ∙ ไทย ∙ Tagalog ∙ toki pona ∙ Türkçe ∙ українська ∙ Oʻzbekcha ∙ vèneto ∙ Tiếng Việt ∙ 中文 ∙ 中文(简体) ∙ 中文(繁體) ∙ +/− |
Описи
Предмети прикажани на податотекава
прикажува
Некоја вредност без предмет на Википодатоци
23 декември 2012
image/gif
Историја на податотеката
Стиснете на датум/време за да ја видите податотеката како изгледала тогаш.
| Датум/време | Минијатура | Димензии | Корисник | Коментар | |
|---|---|---|---|---|---|
| тековна | 22:01, 16 јуни 2021 |  | 480 × 270 (1,6 МБ) | wikimediacommons>TomFryers | Improve render quality and increase resolution and framerate slightly |
Употреба на податотеката
Податотекава се користи во следнава страница: